Formulas are equations that perform calculations on values in your worksheet. A formula starts with an equal sign (=). For example, the following formula multiplies 2 by 3 and then adds 5 to the result.
=5+2*3
A formula can also contain any or all of the following: functions, references, operators, and constants.

Parts of a formula
1. Functions: The PI() function returns the value of pi: 3.142...
2. References: A2 returns the value in cell A2.
3. Constants: Numbers or text values entered directly into a formula, such as 2.
4. Operators: The ^ (caret) operator raises a number to a power, and the * (asterisk) operator multiplies.
In this article
Using constants in formulas
A constant is a value that is not calculated. For example, the date 10/9/2008, the number 210, and the text "Quarterly Earnings" are all constants. An expression, or a value resulting from an expression, is not a constant. If you use constant values in the formula instead of references to the cells (for example, =30+70+110), the result changes only if you modify the formula yourself.
Using calculation operators in formulas
Operators specify the type of calculation that you want to perform on the elements of a formula. There is a default order in which calculations occur, but you can change this order by using parentheses.
Types of operators
There are four different types of calculation operators: arithmetic, comparison, text concatenation, and reference.
Arithmetic operators
To perform basic mathematical operations such as addition, subtraction, or multiplication; combine numbers; and produce numeric results, use the following arithmetic operators.
Arithmetic Operator
|
Meaning
|
Example
|
+ (plus sign)
|
Addition
|
3+3
|
– (minus sign)
|
Subtraction
Negation |
3–1
–1 |
* (asterisk)
|
Multiplication
|
3*3
|
/ (forward slash)
|
Division
|
3/3
|
% (percent sign)
|
Percent
|
20%
|
^ (caret)
|
Exponentiation)
|
3^2
|
Comparison operators
You can compare two values with the following operators. When two values are compared by using these oper
Comparison Operator
|
Meaning
|
Example
|
= (equal sign)
|
Equal to
|
A1=B1
|
> (greater than sign)
|
Greater than
|
A1>B1
|
< (less than sign)
|
Less than
|
A1<B1
|
>= (greater than or equal to sign)
|
Greater than or equal to
|
A1>=B1
|
<= (less than or equal to sign)
|
Less than or equal to
|
A1<=B1
|
<> (not equal to sign)
|
Not equal to
|
A1<>B1
|
ators, the result is a logical value either TRUE or FALSE.
Text concatenation operator
Use the ampersand (&) to join, or concatenate, one or more text strings to produce a single piece of text.
Text Operator
|
Meaning
|
Example
|
& (ampersand)
|
Connects, or concatenates, two values to produce one continuous text value
|
"North"&"wind"
|
Reference operators
Combine ranges of cells for calculations with the following operators.
Reference Operator
|
Meaning
|
Example
|
: (colon)
|
Range operator, which produces one reference to all the cells between two references, including the two references
|
B5:B15
|
, (comma)
|
Union operator, which combines multiple references into one reference
|
SUM(B5:B15,D5:D15)
|
(space)
|
Intersection operator, which produces on reference to cells common to the two references
|
B7:D7 C6:C8
|
The order in which Excel performs operations in formulas
In some cases, the order in which calculation is performed can affect the return value of the formula, so it's important to understand how the order is determined and how you can change the order to obtain desired results.
Calculation order
Formulas calculate values in a specific order. A formula in Excel always begins with an equal sign (=). The equal sign tells Excel that the succeeding characters constitute a formula. Following the equal sign are the elements to be calculated (the operands), which are separated by calculation operators. Excel calculates the formula from left to right, according to a specific order for each operator in the formula.
Operator precedence
If you combine several operators in a single formula, Excel performs the operations in the order shown in the following table. If a formula contains operators with the same precedence — for example, if a formula contains both a multiplication and division operator — Excel evaluates the operators from left to right.
Operator
|
Description
|
: (colon)
(single space)
, (comma)
|
Reference operators
|
–
|
Negation (as in –1)
|
%
|
Percent
|
^
|
Exponentiation
|
* and /
|
Multiplication and division
|
+ and –
|
Addition and subtraction
|
&
|
Connects two strings of text (concatenation)
|
=
< > <= >= <> |
Comparison
|
Use of parentheses
To change the order of evaluation, enclose in parentheses the part of the formula to be calculated first. For example, the following formula produces 11 because Excel calculates multiplication before addition. The formula multiplies 2 by 3 and then adds 5 to the result.
=5+2*3
In contrast, if you use parentheses to change the syntax, Excel adds 5 and 2 together and then multiplies the result by 3 to produce 21.
=(5+2)*3
In the example below, the parentheses around the first part of the formula force Excel to calculate B4+25 first and then divide the result by the sum of the values in cells D5, E5, and F5.
=(B4+25)/SUM(D5:F5)
Using functions and nested functions in formulas
Functions are predefined formulas that perform calculations by using specific values, called arguments, in a particular order, or structure. Functions can be used to perform simple or complex calculations.
The syntax of functions
The following example of the ROUND function rounding off a number in cell A10 illustrates the syntax of a function.
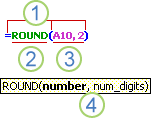
Structure of a function
1. Structure. The structure of a function begins with an equal sign (=), followed by the function name, an opening parenthesis, the arguments for the function separated by commas, and a closing parenthesis.
2. Function name. For a list of available functions, click a cell and press SHIFT+F3.
3. Arguments. Arguments can be numbers, text, logical values such as TRUE or FALSE, arrays, error values such as #N/A, or cell references. The argument you designate must produce a valid value for that argument. Arguments can also be constants, formulas, or other functions.
4. Argument tooltip. A tooltip with the syntax and arguments appears as you type the function. For example, type =ROUND( and the tooltip appears. Tooltips only appear for built-in functions.
Entering functions
When you create a formula that contains a function, the Insert Function dialog box helps you enter worksheet functions. As you enter a function into the formula, the Insert Function dialog box displays the name of the function, each of its arguments, a description of the function and each argument, the current result of the function, and the current result of the entire formula.
To make it easier to create and edit formulas and minimize typing and syntax errors, use formula autocomplete. After you type an = (equal sign) and beginning letters or a display trigger, Microsoft Office Excel displays below the cell a dynamic drop down list of valid functions, arguments, and names that match the letters or trigger. You can then insert an item in the drop-down list into the formula.
Nesting functions
In certain cases, you may need to use a function as one of the arguments of another function. For example, the following formula uses a nested AVERAGE function and compares the result with the value 50.
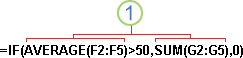
1. The AVERAGE and SUM functions are nested within the IF function.
Valid returns When a nested function is used as an argument, it must return the same type of value that the argument uses. For example, if the argument returns a TRUE or FALSE value, then the nested function must return a TRUE or FALSE. If it doesn't, Microsoft Excel displays a #VALUE! error value.
Nesting level limits A formula can contain up to seven levels of nested functions. When Function B is used as an argument in Function A, Function B is a second-level function. For instance, the AVERAGE function and the SUM function are both second-level functions because they are arguments of the IF function. A function nested within the AVERAGE function would be a third-level function, and so on.
Using references in formulas
A reference identifies a cell or a range of cells on a worksheet and tells Microsoft Excel where to look for the values or data you want to use in a formula. With references, you can use data contained in different parts of a worksheet in one formula or use the value from one cell in several formulas. You can also refer to cells on other sheets in the same workbook, and to other workbooks. References to cells in other workbooks are called links or external references.
The A1 reference style
The default reference style By default, Excel uses the A1 reference style, which refers to columns with letters (A through XFD, for a total of 16,384 columns) and refers to rows with numbers (1 through 1,048,576). These letters and numbers are called row and column headings. To refer to a cell, enter the column letter followed by the row number. For example, B2 refers to the cell at the intersection of column B and row 2.
To Refer To
|
Use
|
The cell in column A and row 10
|
A10
|
The range of cells in column A and rows 10 through 20
|
A10:A20
|
The range of cells in row 15 and columns B through E
|
B15:E15
|
All cells in row 5
|
5:5
|
All cells in rows 5 through 10
|
5:10
|
All cells in column H
|
H:H
|
All cells in columns H through J
|
H:J
|
The range of cells in columns A through E and rows 10 through 20
|
A10:E20
|
Making a reference to another worksheet In the following example, the AVERAGE worksheet function calculates the average value for the range B1:B10 on the worksheet named Marketing in the same workbook.
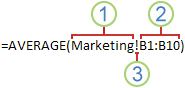
Reference to a range of cells on another worksheet in the same workbook
1. Refers to the worksheet named Marketing
2. Refers to the range of cells between B1 and B10, inclusively
3. Separates the worksheet reference from the cell range reference
The difference between absolute, relative and mixed references
Relative references A relative cell reference in a formula, such as A1, is based on the relative position of the cell that contains the formula and the cell the reference refers to. If the position of the cell that contains the formula changes, the reference is changed. If you copy or fill the formula across rows or down columns, the reference automatically adjusts. By default, new formulas use relative references. For example, if you copy or fill a relative reference in cell B2 to cell B3, it automatically adjusts from =A1 to =A2.
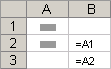
Copied formula with relative reference
Absolute references An absolute cell reference in a formula, such as $A$1, always refer to a cell in a specific location. If the position of the cell that contains the formula changes, the absolute reference remains the same. If you copy or fill the formula across rows or down columns, the absolute reference does not adjust. By default, new formulas use relative references, and you may need to switch them to absolute references. For example, if you copy or fill an absolute reference in cell B2 to cell B3, it stays the same in both cells =$A$1.

Copied formula with absolute reference
Mixed references A mixed reference has either an absolute column and relative row, or absolute row and relative column. An absolute column reference takes the form $A1, $B1, and so on. An absolute row reference takes the form A$1, B$1, and so on. If the position of the cell that contains the formula changes, the relative reference is changed, and the absolute reference does not change. If you copy or fill the formula across rows or down columns, the relative reference automatically adjusts, and the absolute reference does not adjust. For example, if you copy or fill a mixed reference from cell A2 to B3, it adjusts from =A$1 to =B$1.

Copied formula with mixed reference
The 3-D reference style
Conveniently referencing multiple worksheets If you want to analyze data in the same cell or range of cells on multiple worksheets within the workbook, use a 3-D reference. A 3-D reference includes the cell or range reference, preceded by a range of worksheet names. Excel uses any worksheets stored between the starting and ending names of the reference. For example, =SUM(Sheet2:Sheet13!B5) adds all the values contained in cell B5 on all the worksheets between and including Sheet 2 and Sheet 13.
- You can use 3-D references to refer to cells on other sheets, to define names, and to create formulas by using the following functions: SUM, AVERAGE, AVERAGEA, COUNT, COUNTA, MAX, MAXA, MIN, MINA, PRODUCT, STDEV, STDEVA, STDEVP, STDEVPA, VAR, VARA, VARP, and VARPA.
- 3-D references cannot be used in array formulas.
- 3-D references cannot be used with the intersection operator (a single space) or in formulas that use implicit intersection.
What happens when you move, copy, insert, or delete worksheets The following examples explain what happens when you move, copy, insert, or delete worksheets that are included in a 3-D reference. The examples use the formula =SUM(Sheet2:Sheet6!A2:A5) to add cells A2 through A5 on worksheets 2 through 6.
- Insert or copy If you insert or copy sheets between Sheet2 and Sheet6 (the endpoints in this example), Microsoft Excel includes all values in cells A2 through A5 from the added sheets in the calculations.
- Delete If you delete sheets between Sheet2 and Sheet6, Excel removes their values from the calculation.
- Move If you move sheets from between Sheet2 and Sheet6 to a location outside the referenced sheet range, Excel removes their values from the calculation.
- Move an endpoint If you move Sheet2 or Sheet6 to another location in the same workbook, Excel adjusts the calculation to accommodate the new range of sheets between them.
- Delete an endpoint If you delete Sheet2 or Sheet6, Excel adjusts the calculation to accommodate the range of sheets between them.
The R1C1 reference style
You can also use a reference style where both the rows and the columns on the worksheet are numbered. The R1C1 reference style is useful for computing row and column positions in macros. In the R1C1 style, Excel indicates the location of a cell with an "R" followed by a row number and a "C" followed by a column number.
Reference
|
Meaning
|
R[-2]C
|
A relative reference to the cell two rows up and in the same column
|
R[2]C[2]
|
A relative reference to the cell two rows down and two columns to the right
|
R2C2
|
An absolute reference to the cell in the second row and in the second column
|
R[-1]
|
A relative reference to the entire row above the active cell
|
R
|
An absolute reference to the current row
|
When you record a macro, Excel records some commands by using the R1C1 reference style. For example, if you record a command such as clicking the AutoSum button to insert a formula that adds a range of cells, Excel records the formula by using R1C1 style, not A1 style, references.
You can turn the R1C1 reference style on or off by setting or clearing the R1C1 reference style check box under the Working with formulas section in the Formulas category of the Excel Settings dialog box that you display from the Microsoft Office Button  .
.
 .
.Using names in formulas
You can create defined names to represent cells, ranges of cells, formulas, constant values, or Excel tables. A name is a meaningful shorthand that makes it easier to understand the purpose of a cell reference, constant, formula, or table, each of which may be difficult to comprehend at first glance. The following information shows common examples of names and how they can improve clarity and understanding.
Example Type
|
Example With No Name
|
Example With A Name
|
Reference
|
=SUM(C20:C30)
|
=SUM(FirstQuarterSales)
|
Constant
|
=PRODUCT(A5,8.3)
|
=PRODUCT(Price,WASalesTax)
|
Formula
|
=SUM(VLOOKUP(A1,B1:F20,5,FALSE), —G5)
|
=SUM(Inventory_Level,—Order_Amt)
|
Table
|
C4:G36
|
=TopSales06
|
Types of names
There are several types of names you can create and use.
Defined name A name that represents a cell, range of cells, formula, or constant value. You can create your own defined name, and Excel sometimes creates a defined name for you, such as when you set a print area.
Table name A name for an Excel table, which is a collection of data about a particular subject that is stored in records (rows) and fields (columns). Excel creates a default Excel table name of "Table1", "Table2", and so on, each time you insert an Excel table, but you can change the name to make it more meaningful. For more information on Excel tables, see Using structured references with Excel tables.
Creating and entering names
You create a name by using the:
- Name box on the formula bar This is best used for creating a workbook level name for a selected range.
- Create a name from selection You can conveniently create names from existing row and column labels by using a selection of cells in the worksheet.
- New Name dialog box This is best used for when you want more flexibility in creating names, such as specifying a local worksheet level scope or creating a name comment.
NOTE: By default, names use absolute cell references.
You can enter a name by:
- Typing Typing the name, for example, as an argument to a formula.
- Using Formula AutoComplete Use the Formula AutoComplete drop-down list, where valid names are automatically listed for you.
- Selecting from the Use in Formula command Select a defined name from a list available from the Use in Formulacommand in the Defined Names group on the Formula tab.
For more information, see Use names to clarify formulas.
Using array formulas and array constants
An array formula can perform multiple calculations and then return either a single result or multiple results. Array formulas act on two or more sets of values known as array arguments. Each array argument must have the same number of rows and columns. You create array formulas in the same way that you create other formulas, except you press CTRL+SHIFT+ENTER to enter the formula. Some of the built-in functions are array formulas, and must be entered as arrays to get the correct results.
Array constants can be used in place of references when you don't want to enter each constant value in a separate cell on the worksheet.
Using an array formula to calculate single and multiple results
When you enter an array formula, Microsoft Excel automatically inserts the formula between { } (braces).
To calculate a single result This type of array formula can simplify a worksheet model by replacing several different formulas with a single array formula.
For example, the following calculates the total value of an array of stock prices and shares, without using a row of cells to calculate and display the individual values for each stock.
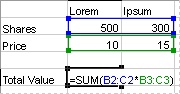
Array formula that produces a single result
When you enter the formula ={SUM(B2:D2*B3:D3)} as an array formula, it multiples the Shares and Price for each stock, and then adds the results of those calculations together.
To calculate multiple results Some worksheet functions return arrays of values, or require an array of values as an argument. To calculate multiple results with an array formula, you must enter the array into a range of cells that has the same number of rows and columns as the array arguments.
For example, given a series of three sales figures (in column B) for a series of three months (in column A), the TREND function determines the straight-line values for the sales figures. To display all of the results of the formula, it is entered into three cells in column C (C1:C3).

Array formula that produces multiple results
When you enter the formula =TREND(B1:B3,A1:A3) as an array formula, it produces three separate results (22196, 17079, and 11962), based on the three sales figures and the three months.
Using array constants
In an ordinary formula, you can enter a reference to a cell containing a value, or the value itself, also called a constant. Similarly, in an array formula you can enter a reference to an array, or enter the array of values contained within the cells, also called an array constant. Array formulas accept constants in the same way that nonarray formulas do, but you must enter the array constants in a certain format.
Array constants can contain numbers, text, logical values such as TRUE or FALSE, or error values such as #N/A. Different types of values can be in the same array constant — for example, {1,3,4;TRUE,FALSE,TRUE}. Numbers in array constants can be in integer, decimal, or scientific format. Text must be enclosed in double quotation marks — for example, "Tuesday".
Array constants cannot contain cell references, columns or rows of unequal length, formulas, or the special characters $ (dollar sign), parentheses, or % (percent sign).
When you format array constants, make sure you:
- Enclose them in braces ( { } ).
- Separate values in different columns with commas (,). For example, to represent the values 10, 20, 30, and 40, enter {10,20,30,40}. This array constant is known as a 1-by-4 array and is equivalent to a 1-row-by-4-column reference.
- Separate values in different rows with semicolons (;). For example, to represent the values 10, 20, 30, and 40 in one row and 50, 60, 70, and 80 in the row immediately below, you would enter a 2-by-4 array constant: {10,20,30,40;50,60,70,80}.